This paper studies the trajectory asymptotic behavior of solutions for equations of a class of three-dimensional autonomous and incompressible non-Newtonian fluids. The authors prove the existence of the trajectory attractor for this class of equations in an appropriate topological space.

This paper is concerned with the stability of traveling wave fronts for reaction diffusion equations with nonlocal delay. We prove that, in the appropriate weighted L^∞ spaces, the non-critical traveling wave fronts are globally exponentially stable, and the critical traveling wave fronts are globally algebraically stable. Moreover, we obtain the rates of convergence by weighted energy estimates. We apply these results to a host-vector disease model, the generalized Nicholson blowflies equation, and a modified vector disease model.

We mainly prove the boundedness of the commutators of Hardy type operators on Herz spaces , where α(·) and p(·) are both variable.

Using maximum modulus principle, we investigate the problem of the existence of the transcendental meromorphic solutions of system of complex algebraic differential equations, and obtain a result which the system only the algebraic solution under certain conditions. Examples show that our results are sharp.

We characterize two subclasses of quasisymmetric homeomorphisms of the unit circle in terms of higher order Schwarzian derivatives, which are the strongly symmetric homeomorphisms and the Weil-Petersson class.

Super O-operators on Pre-Lie superalgebras are introduced. The solutions of super S-equation are discussed by super O-operators. Then the notion of L-dendriform superalgebras is introduced as the algebraic structure behind the super O-operators. Moreover, the relation between L-dendriform superalgebras, dendriform superalgebras and Pre-Lie superalgebra is also established.

Let H and K be complex Hilbert spaces. Let A and B be two factor von Neumann algebras acting on H and K respectively. A characterization of *-isomorphism between A and B is given. Let Φ: A → B be a bijection. If Φ(A^*B + B^*A) = Φ(A)^*Φ(B) + Φ(B)^*Φ(A) for all A,B ∈ A, then Φ is a linear or a conjugate linear *-isomorphism.

We consider the count of the coming generation of subcritical CMJ processes in discrete time. With the help of several renewal theorems, we obtain the limit theorems for the conditional processes. Therefore, we show how the coming generation sheds new light on the limit properties of subcritical CMJ processes.

We provide a characterization of wave packet Parseval frames from their Fourier transforms, which deduces some existing results as e corollaries. Furthermore, some properties and a sufficient condition of wave packet frame multipliers are obtained.

It estimates the volume of an area-bounded minimal graph supported on Ω which is called admissible and it has Euclidean growth. This result generalizes the related results of Elbert and Rosenberg in Mⁿ×R for minimal graphs to in Mⁿ×R^m.

We show new estimates of multilinear multipliers, improve the known results. We apply the results obtained into the estimates of the nonlinearities of differential equations.

Given a finite-dimensional algebra A over a field k, and a quiver with relations (Q,I), let Λ = A⊕_kkQ/I. The category Λ-Mod (Resp. Λ-mod) of (Resp. finitely generated) Λ-modules is equivalent to the category Rep(Q, I,A) (Resp. rep(Q, I,A)) of (Resp. finite-dimensional) representations of (Q, I) over A. 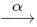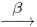The main result of this paper explicitly describes the Gorenstein-projective modules in rep(A₃,I,A), where (A₃, I) = 3 α 21. with relation I = < βα >. As a corollary, we give a necessary and sufficient condition for A being a self-injective algebra.

Multiplication operator is an important class of operators in function space. We mainly research the properties of the multiplication operator on the weighted Bergman space of the unit ball.

We show that both exponential energy and exponentially harmonic map are conformally invariant under a circumstance. We investigate exponentially harmonic Riemannian submersions and exponentially harmonic isometric immersions. We also examine the associated Gauss map of a Riemannian isometric immersion which is exponentially harmonic.

We study the asymptotic estimates of unimodular multipliers on α modulation spaces. We make a unified study about different types of function spaces, including modulation spaces and Besov spaces. The upper bound estimates of our results generalize many known results of modulation spaces. Moreover, under a partial non-degenerate assumptions on the symbol, we get the sharp asymptotic estimates of the unimodular Fourier multipliers.

We give the necessary conditions of boundedness of multilinear Hausdorff operators on Lebesgue spaces and λ-central Morrey spaces, respectively, when the kernel functions are nonnegative. Meanwhile, the corresponding operator norms are worked out.

We study the strongly damped wave equations with critical nonlinearities. By choosing suitable state spaces, we prove sectorial properties of the operator matrix , analysis the criticality of the nonlinearities with critical growth, and investigate the local and global existence of weak solutions together with their higher regularity.

We mainly discuss the complex convexity of Musielak-Orlicz sequence spaces equipped with the p-Amemiya norm. It is obtained that for any Musielak- Orlicz sequence space equipped with the p-Amemiya norm when 1 ≤ p < ∞, complex strongly extreme points of the unit ball coincide with complex extreme points of the unit ball. Moreover, criteria for them in above spaces are given. Criteria for complex rotundity and complex mid-point locally uniform convexity of above spaces are also deduced.

We estimate the crossing number of framed link in thickened surface, we define the span of framed link in Kauffman bracket skein module, which may give a lower bound of the crossing number.

Our first aim in this paper is to construct a modified Green function in a cone and give the growth property of it. As an application, we next give the general solutions of the Dirichlet problem. Finally, we prove that this solution is unique.

An umbilical free oriented hypersurface x:M→Rⁿ with nonzero princi-pal curvatures is called Laguerre isoparametric hypersurface if its Laguerre form C=∑_i C_iw_i =∑_iρ^-1(E_i(logρ)(r-r_i)-E_i(r))w_i vanishes and Laguerre shape operator S=ρ^-1(S-rid has constant eigenvalues. Here ρ²=∑_i(r-r_i)²,r=r₁+r₂+…+r_n-1/n-1 is the mean curvature radius and S is the shape operator of x, E_i is local orthogonal basis for Laguerre metric g with dual basis w_i. In this paper, we classify all Laguerre isoprametric hypersurfaces in Rⁿ with three distinct Laguerre principal curvatures up to Laguerre transformation.

We propose an algorithms for finding a common fixed point of a finite family of multi-valued Bregman total quasi-φ-asymptotically nonexpansive mappings in reflexive Banach spaces. The results presented in the paper improve and extend the corresponding new results announced by many authors. The algorithms are based on the Bregman distance related to a well-chosen convex function. As applications, we apply our results to a system of equilibrium problems and zero point problem of maximal monotone mappings in reflexive Banach spaces.

This paper is devoted to the large time behavior and especially to the regularity of the global attractor for the semi-discrete in time Crank-Nicolson scheme to discretize a class of system of nonlinear Schrödinger-BBM equations on R × R. We first prove that such a semi-discrete system provides a discrete infinite-dimensional dynamical system in H¹ × H¹ that possesses a global attractor A_τ in H¹ × H¹. We also show that the global attractor A_τ is regular, i.e. A_τ is actually included, bounded and compact in H^(3/2)-ε×H².

Algebraic extension of nth root of class A operator is studied. Specifically, we obtain that every algebraic extension of nth root of class A operator is subscalar. The proof uses the space decomposition technique. As a consequence, we consider the Weyl type theorems and the hyperinvariant subspace for such operator.

We obtain the general solution and stability of the Jensen-quadratic functional equation f((x₁+x₂)/2,y₁+y₂)+f((x₁+x₂)/2,y₁-y₂)=f(x₁,y₁)+f(x₁,y₂)+f(x₂,y₁)+f(x₂,y₂).

We consider the existence of positive solutions to the following semiposi-tone (k,n-k) conjugate boundary value problems (SCBVP): 

where n≥2, 1<k<n-1. The nonlinear function f may change sign for 0 < t < 1, i.e., we allow that the nonlinear term f is both semipositone and lower unbounded. Without making any monotone-type assumption, by using the fixed-point index theory, the existence of positive solution and many positive solutions are obtained.

This paper focus on the L^p estimates of the higher order damped wave equation. Using tools from harmonic analysis, especially the method of oscillatory integral, we calculated the point-wise estimates for the kernel related to the solution of the homogeneous equation. Also we apply these estimates to study the space-time estimates and the global solution to the Cauchy problem of the nonlinear equation.

Firstly, the concept of upper covering relation in a union semi-lattice and upper coverage defined by the forme in an enriched union semi-lattice are introduced, the basic properties of them are discussed in enriched union semi-lattices. Secondly, the concept of C-filters is introduced in enriched union semi-lattices through upper coverage, it is proved that C-filters are usual filters, but usual filters are not C-filters in an enriched union semi-lattice. Thirdly, the relationships between morphisms of enriched union semi-lattice and co-Frames are investigated. At last, the isomorphism theorem between co-Frames and its C_S-filter co-Frames is proved.

Let F be a field and char F = p > 3. Let g denote the Lie superalgebra which is the tensor product of an exterior superalgebra and a finite-dimensional generalized Witt algebra or special Lie algebra over F. In this paper it is proved that the standard filtration of the non-simple Lie superalgebra g is invariant under the automorphism group of g by means of investigating the ad nilpotent elements. Finally, the superalgebras of type W or S are classified by means of the parameters defining them.

In this paper, the authors establish some boundedness of homogeneous fractional integrals on some variable exponent function spaces, such as variable Lebesgue spaces, variable Hardy spaces and variable Herz-type Hardy spaces.

Let G be a group. A subset X in G is said to be non-commuting if xy≠yx for any x, y ∈ X with x ≠y. Furthermore, if |X| ≥ |Y| for any other non-commuting subset Y in G, then X is said to be a maximal non-commuting set. In this paper, the bound for the cardinality of a maximal non-commuting set in a finite p-group with a cyclic center is determined.

Affine factorable surfaces in Euclidean 3-space were defined. The classification of surfaces with zero Gauss curvature and nonzero constant mean curvature and minimal affine factorable surfaces was given.

We determine and estimate the Ptolemy constant of absolute normalized norms on some new conditions. The new results which not only contain the previous results, but also give some new results in some concrete Banach spaces.

Let F be a family of meromorphic functions in a domain D, let a(z), b(z), c(z) be three distinct meromorphic functions in D, one may be ∞, and for any z∈ D, a(z)= b(z), a(z) = c(z), b(z) = c(z), and let S = {a(z), b(z), c(z)}. If for any two functions f, g∈F, f and g share the set S in D, then F is normal in D. This extends the famous Montel's criterion.

We investigate the irreducibility of Verma modules over the completed Witt algebra. By embedding the Virasoro algebra into the completed Witt algebra, we give realizations of two classes of irreducible non-weight Virasoro-modules. We also obtain some interesting combinatorial identities.

Let G be finite group such that M(G) = M(²G₂(3²ⁿ⁺¹)). Then G has a normal subgroup isomorphic to ²G₂(3²ⁿ⁺¹). Especially, if |G| = |²G₂(3²ⁿ⁺¹)|, then G ≌²G₂(3²ⁿ⁺¹).

Let F be an algebraically closed field of characteristic p > 3. Using the invariance of the natural filtration and calculating the actions on the -1 components for the Hom-structures of the modular Lie superalgebras under consideration, we prove that there exists only the trivial multiplicative Hom-structures on the finite dimensional Z-graded modular Lie superalgebras of Cartan type.

We present some explicit formulae for genus of the composite field of Kummer and Artin-Schreier function fields in some specific cases. Meanwhile, we point out in this note that the genus of function field we addressed reaches the upper bound of Castelnuovo's inequality.

The infinite-dimensional Lie superalgebras of L-type over a field of positive characteristic are studied. The simplicity is proved and the natural filtration of Lie superalgebras of L-type is proved to be invariant under automorphism group by using ad-nilpotent elements. We are thereby able to obtain a property of Lie superalgebras of L-type.

We classify the general symmetric operator algebras on Pontrjagin space into six classes by using of symmetric ideals, and give the general forms for all the SM operator algebras in each class.